Electromagnetism and electromagnetic induction shape the world around us. These principles power technologies like electric vehicles, cellular phones, and spacecraft. Electromagnetic theory forms the backbone of modern physics. Data in ebook gadgets and phones rely on electromagnetic formats for storage. The significance of electromagnetism extends to everyday life. Three key principles guide these phenomena: Faraday’s Law, Lenz’s Law, and Magnetic Flux. Each principle plays a vital role in the functionality of numerous devices. Understanding these principles enhances our grasp of technology’s impact on daily life.
Historical Background
The journey of electromagnetism began in the late 18th century. Scientists like Coulomb and Gauss developed mathematical laws to explain electromagnetic interactions. In 1820, Hans C. Ørsted discovered that electric currents produce magnetic fields. This discovery led Ampère to explore electrodynamics further. Michael Faraday made a groundbreaking discovery in 1831. He showed that changing magnetic fields could induce currents. This discovery laid the foundation for modern electric power. James Clerk Maxwell later described this phenomenon mathematically as Faraday’s law of induction. The 1860s saw the formulation of Maxwell’s equations. These equations predicted electromagnetic waves, revolutionizing communication technology.
Understanding Electromagnetism and Electromagnetic Induction
Basic Concepts of Electromagnetism and Electromagnetic Induction
Definition and Importance
Electromagnetism involves the interaction between electric charges and magnetic fields. This interaction forms the basis for many technologies. Electromagnetic induction occurs when a conductor moves through a magnetic field. This movement generates an electromotive force (emf). The principles of electromagnetism power devices like motors and generators. Understanding these concepts helps us grasp how technology impacts daily life.
Electromagnetic Forces
Electric Fields and Magnetic Fields
Electric fields arise from charged particles. These fields exert forces on other charges. Magnetic fields result from moving charges or magnets. These fields influence magnetic materials and moving charges. Both fields play crucial roles in electromagnetic interactions.
Interaction between Charges and Fields
Charges interact with electric and magnetic fields in fascinating ways. A charge in an electric field experiences a force. This force can cause the charge to move. A moving charge in a magnetic field also experiences a force. This interaction forms the basis for many technologies. Devices like electric motors rely on these principles to function effectively. Understanding these interactions enhances our appreciation of technology’s role in our lives.
Key Concepts
- Electromagnetic Induction: This phenomenon occurs when a conductor experiences a change in magnetic flux, leading to the generation of an electric current. The induced current flows in a direction that opposes the change in magnetic flux, as described by Lenz’s Law.
- Magnetic Flux: Magnetic flux is defined as the product of the magnetic field and the area through which the field lines pass, taking into account the angle between the field lines and the normal to the surface:
Magnetic Flux
Before studying electromagnetism, we must have a solid understanding of magnetic flux.
Definition and Explanation
What is Magnetic Flux?
Magnetic Flux, 
Magnetic Flux Concept
The concept helps in understanding how magnetic fields interact with materials. A strong magnetic field results in a high magnetic flux. Engineers use this principle to design devices that rely on magnetic fields. Magnetic flux measures the quantity of magnetic field lines passing through a given area.
It is an important concept in electromagnetism, particularly in the context of electromagnetic induction. The magnetic flux through a surface is defined mathematically as:
![\[\Phi_B = \int \mathbf{B} \cdot d\mathbf{A}\]](https://images.rapidload-cdn.io/spai/ret_blank,q_lossy,to_avif/https://entechonline.com/wp-content/ql-cache/quicklatex.com-1a8274d35f77883e7b9af1de199338c7_l3.png "Rendered by QuickLaTeX.com")
where:
The dot product (⋅) indicates that only the component of the magnetic field perpendicular to the surface contributes to the flux.
For a uniform magnetic field and a flat surface, the magnetic flux can be expressed as:
![\[\Phi_B = B \cdot A \cdot \cos(\theta)\]](https://images.rapidload-cdn.io/spai/ret_blank,q_lossy,to_avif/https://entechonline.com/wp-content/ql-cache/quicklatex.com-176a44c4782e85a5fcb8cd05a9d861f1_l3.png "Rendered by QuickLaTeX.com")
where:
Example Problem
Problem Statement
Solution
1. Calculate the Area of the Loop: The area A of a circle is given by the formula:
2. Calculate the Magnetic Flux: Since the magnetic field is perpendicular to the surface of the loop, the angle θ between the magnetic field and the normal to the surface is 0 deg. Thus, we can use the simplified formula for magnetic flux:
Real-Life Applications
MRI Machines
MRI machines use magnetic flux to create detailed images of the human body. The machine generates a strong magnetic field. This field interacts with hydrogen atoms in the body. The interaction produces signals that the machine converts into images. Doctors use these images to diagnose medical conditions accurately.
Transformers
Transformers rely on magnetic flux to transfer electrical energy between circuits. The device consists of coils wrapped around a core. An alternating current in one coil creates a changing magnetic field. This field induces a voltage in the other coil. Engineers use transformers to adjust voltage levels for efficient power distribution.
Faraday’s Law of Electromagnetic Induction
Definition and Explanation
Faraday’s Law Statement
Faraday’s Law reveals a fascinating concept. A changing magnetic field induces an electromotive force (emf) in a circuit. This principle forms the foundation of electromagnetic induction. Michael Faraday discovered this relationship in 1831. The law states that the magnitude of the induced emf is proportional to the rate of change of magnetic flux.
Mathematical Representation:
(1) 
where:
Example Problem
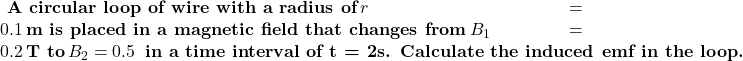
Real-Life Applications
Electric Generators
Electric generators rely on Faraday’s Law. Rotating coils within a magnetic field produce electricity. This process powers homes and industries. Generators convert mechanical energy into electrical energy efficiently.
Induction Cooktops
Induction cooktops use electromagnetic induction. A coil beneath the cooking surface generates a magnetic field. This field induces currents in the cookware. The cookware heats up, cooking food quickly and evenly. Induction cooktops offer energy efficiency and safety.
Lenz’s Law
Definition and Explanation
Lenz’s Law Statement
Lenz’s Law states that the direction of the induced electromotive force (emf) opposes the change in magnetic flux that causes it. This principle ensures that the induced current creates a magnetic field opposing the initial change. Lenz’s Law helps maintain energy conservation in electromagnetic systems.
Example Problem
Since the magnetic field is decreasing, the induced current will flow in a direction that tries to maintain the magnetic field inside the loop. If the original magnetic field is directed into the page, the induced current will flow in a counterclockwise direction.
Real-Life Applications
Braking Systems in Trains
Braking systems in trains utilize Lenz’s Law. When a train slows down, the braking system generates a magnetic field. This field induces currents in the wheels, creating resistance. The resistance opposes the train’s motion, slowing it down effectively. Lenz’s Law ensures smooth and efficient braking.
Metal Detectors
Metal detectors rely on Lenz’s Law for operation. A metal detector emits a magnetic field. When metal objects enter this field, they induce currents. These currents create their own magnetic fields, which the detector senses. Lenz’s Law helps identify metal objects by detecting these changes in magnetic fields.
Integration of Principles
How They Work Together
Interrelation of Faraday’s and Lenz’s Laws
Faraday’s Law and Lenz’s Law form a powerful duo in electromagnetism and electromagnetic induction. Faraday’s Law states that a changing magnetic field induces an electromotive force (emf) in a conductor. Lenz’s Law adds depth to this concept. Lenz’s Law explains that the induced current will oppose the change in magnetic flux that caused it. The negative sign in Faraday’s Law formula represents this opposition. This relationship ensures energy conservation in electromagnetic systems. Engineers use this understanding to design efficient devices.
Role in Electromagnetic Devices
Electromagnetic devices rely on the integration of these principles. Electric generators convert mechanical energy into electrical energy. The rotating coils in a magnetic field induce an emf, following Faraday’s Law. Lenz’s Law ensures that the induced current opposes the motion, maintaining energy balance. Transformers adjust voltage levels for power distribution. The alternating current in one coil creates a changing magnetic field. This field induces a voltage in another coil, demonstrating Faraday’s Law. Lenz’s Law ensures efficient energy transfer by opposing unnecessary changes. These principles guide the design and operation of countless technologies.
Electromagnetism and electromagnetic induction shape our world. Faraday’s Law, Lenz’s Law, and Magnetic Flux are key principles. These principles power technologies like electric generators and MRI machines. Electromagnetism plays a crucial role in everyday life. Understanding these concepts enhances our appreciation of modern technology. Exploring these principles further can reveal fascinating insights into how devices work. The study of electromagnetism informs the construction of electric circuits and semiconductor devices. This exploration can inspire innovation and deepen our knowledge of the universe.
To stay updated with the latest developments in STEM research, visit ENTECH Online. This is our digital magazine for science, technology, engineering, and mathematics.
At ENTECH Online, you’ll find a wealth of information. We offer insights and resources to fuel your curiosity. Our goal is to inspire your passion for new scientific discoveries.
Image Source: unsplash
Disclaimer
This article/blog post is not intended to provide professional or technical or medical advice. Please consult with a healthcare professional before making any changes to your diet or lifestyle. AI-generated images are used only for illustration and decoration. Their accuracy, quality, and appropriateness can differ. Users should avoid making decisions or assumptions based only on the text and images.
